- 著者
- E. Sakai
- 出版者
- THE PHYSICAL SOCIETY OF JAPAN , The Mathematical Society of Japan
- 雑誌
- Tokyo Sugaku-Butsurigaku Kwai Kiji (ISSN:21852669)
- 巻号頁・発行日
- vol.8, no.1, pp.13-18, 1896 (Released:2010-03-16)
1 0 0 0 OA HONKWAIKIZI
- 出版者
- THE PHYSICAL SOCIETY OF JAPAN , The Mathematical Society of Japan
- 雑誌
- Tokyo Sugaku-Butsurigaku Kwai Kiji (ISSN:21852669)
- 巻号頁・発行日
- vol.8, no.2, pp.47-51, 1896 (Released:2010-03-16)
- 著者
- Shunkichi Kimura
- 出版者
- THE PHYSICAL SOCIETY OF JAPAN , The Mathematical Society of Japan
- 雑誌
- Tokyo Sugaku-Butsurigaku Kwai Kiji (ISSN:21852669)
- 巻号頁・発行日
- vol.8, no.2, pp.53-89, 1896 (Released:2010-03-16)
- 著者
- K. Tsuruta
- 出版者
- THE PHYSICAL SOCIETY OF JAPAN , The Mathematical Society of Japan
- 雑誌
- Tokyo Sugaku-Butsurigaku Kwai Kiji (ISSN:21852669)
- 巻号頁・発行日
- vol.7, no.4, pp.57-59, 1896 (Released:2010-03-16)
1 0 0 0 OA NOTE ON A GEOMETRICAL PROBLEM
- 著者
- T. Hayashi
- 出版者
- THE PHYSICAL SOCIETY OF JAPAN , The Mathematical Society of Japan
- 雑誌
- Tokyo Sugaku-Butsurigaku Kwai Kiji (ISSN:21852669)
- 巻号頁・発行日
- vol.7, no.4, pp.60-64, 1896 (Released:2010-03-16)
- 参考文献数
- 1
- 著者
- Riemann
- 出版者
- THE PHYSICAL SOCIETY OF JAPAN , The Mathematical Society of Japan
- 雑誌
- Tokyo Sugaku-Butsurigaku Kwai Kiji (ISSN:21852669)
- 巻号頁・発行日
- vol.7, no.4, pp.65-78, 1896 (Released:2010-03-16)
PLAN of the Inquiry:I. Notion of an n-ply extended magnitude.*§1. Continuous and discrete manifoldnesses. Defined parts of a manifoldness are called Quanta. Division of the theory of continuous magnitude into the theories, (1) Of mere region-relations, in which an independence of magnitudes from position is not assumed;(2) Of size-relations, in which such an independence must be assumed.§2. Construction of the notion of a one-fold, two-fold, n-fold extended magnitude.§3. Reduction of place-fixing in a given manifoldness to quantityfixings. True character of an n-fold extended magnitude.II. Measure-relations of which a manifoldness of n-dimensions is capable on the assumption that lines have a length independent of position, and consequently that every line may be measured by every other.**§1. Expression for the line-element. Manifoldnesses to be called Flat in which the line-element is expressible as the square root of a sum of squares of complete differentials.§2. Investigation of the manifoldness of n-dimensions in which the line-element may be represented as the square root of a quadric differential. Measure of its deviation from flatness (curvature) at a given point in a given surface-direction. For the determination of its measure-relations it is allowable and sufficient that the curvature be arbitrarily given at every point in 1/2 n(n-1) surface directions.§3. Geometric illustration.§4. Flat manifoldnesses (in which the curvature is everywhere=o) may be treated as a special case of manifoldnesses with constant curvature. These can also be defined as admitting an independence of n-fold extents in them from position (possibility of motion without stretching).§5. Surfaces with constant curvature.III. Application to Space.§1. System of facts which suffice to-determine the measure-relations of space assumed in geometry.§2. How far is the validity of these empirical determinations probable beyond the limits of observation towards the infinitely great?§3. How far towards the infinitely small? Connection of this question with the interpretation of nature.***
1 0 0 0 OA HONKWAI KIJI 5
- 出版者
- THE PHYSICAL SOCIETY OF JAPAN , The Mathematical Society of Japan
- 雑誌
- Tokyo Sugaku-Butsurigaku Kwai Kiji (ISSN:21852669)
- 巻号頁・発行日
- vol.7, no.5, pp.79-80, 1896 (Released:2010-03-16)
- 著者
- D. Kikuchi
- 出版者
- THE PHYSICAL SOCIETY OF JAPAN , The Mathematical Society of Japan
- 雑誌
- Tokyo Sugaku-Butsurigaku Kwai Kiji (ISSN:21852669)
- 巻号頁・発行日
- vol.7, no.5, pp.107-110, 1896 (Released:2010-03-16)
- 参考文献数
- 4
1 0 0 0 OA HONKWAI KIJI 6
- 出版者
- THE PHYSICAL SOCIETY OF JAPAN , The Mathematical Society of Japan
- 雑誌
- Tokyo Sugaku-Butsurigaku Kwai Kiji (ISSN:21852669)
- 巻号頁・発行日
- vol.7, no.6, pp.111-113, 1896 (Released:2010-03-16)
- 著者
- D. Kikuchi
- 出版者
- THE PHYSICAL SOCIETY OF JAPAN , The Mathematical Society of Japan
- 雑誌
- Tokyo Sugaku-Butsurigaku Kwai Kiji (ISSN:21852669)
- 巻号頁・発行日
- vol.7, no.6, pp.114-117, 1896 (Released:2010-03-16)
1 0 0 0 OA HONKWAI KIJI 2
- 出版者
- THE PHYSICAL SOCIETY OF JAPAN , The Mathematical Society of Japan
- 雑誌
- Tokyo Sugaku-Butsurigaku Kwai Kiji (ISSN:21852669)
- 巻号頁・発行日
- vol.7, no.2, pp.19-23, 1896 (Released:2010-03-16)
- 著者
- D. Kikuchi
- 出版者
- THE PHYSICAL SOCIETY OF JAPAN , The Mathematical Society of Japan
- 雑誌
- Tokyo Sugaku-Butsurigaku Kwai Kiji (ISSN:21852669)
- 巻号頁・発行日
- vol.7, no.2, pp.24-26, 1896 (Released:2010-03-16)
1 0 0 0 OA HONKWAI KIJI 3
- 出版者
- THE PHYSICAL SOCIETY OF JAPAN , The Mathematical Society of Japan
- 雑誌
- Tokyo Sugaku-Butsurigaku Kwai Kiji (ISSN:21852669)
- 巻号頁・発行日
- vol.7, no.3, pp.31-32, 1896 (Released:2010-03-16)
- 著者
- Fujioka Ichisuke
- 出版者
- THE PHYSICAL SOCIETY OF JAPAN , The Mathematical Society of Japan
- 雑誌
- Tokyo Sugaku-Butsurigaku Kwai Kiji (ISSN:21852669)
- 巻号頁・発行日
- vol.7, no.3, pp.33-38, 1896 (Released:2010-03-16)
- 著者
- K. Tsuruta
- 出版者
- THE PHYSICAL SOCIETY OF JAPAN , The Mathematical Society of Japan
- 雑誌
- Tokyo Sugaku-Butsurigaku Kwai Kiji (ISSN:21852669)
- 巻号頁・発行日
- vol.7, no.3, pp.39-46, 1896 (Released:2010-03-16)
- 著者
- D. Kikuchi
- 出版者
- THE PHYSICAL SOCIETY OF JAPAN , The Mathematical Society of Japan
- 雑誌
- Tokyo Sugaku-Butsurigaku Kwai Kiji (ISSN:21852669)
- 巻号頁・発行日
- vol.7, no.3, pp.47-53, 1896 (Released:2010-03-16)
- 参考文献数
- 8
1 0 0 0 OA HONKWAI KIJI 4
- 出版者
- THE PHYSICAL SOCIETY OF JAPAN , The Mathematical Society of Japan
- 雑誌
- Tokyo Sugaku-Butsurigaku Kwai Kiji (ISSN:21852669)
- 巻号頁・発行日
- vol.7, no.4, pp.55-56, 1896 (Released:2010-03-16)
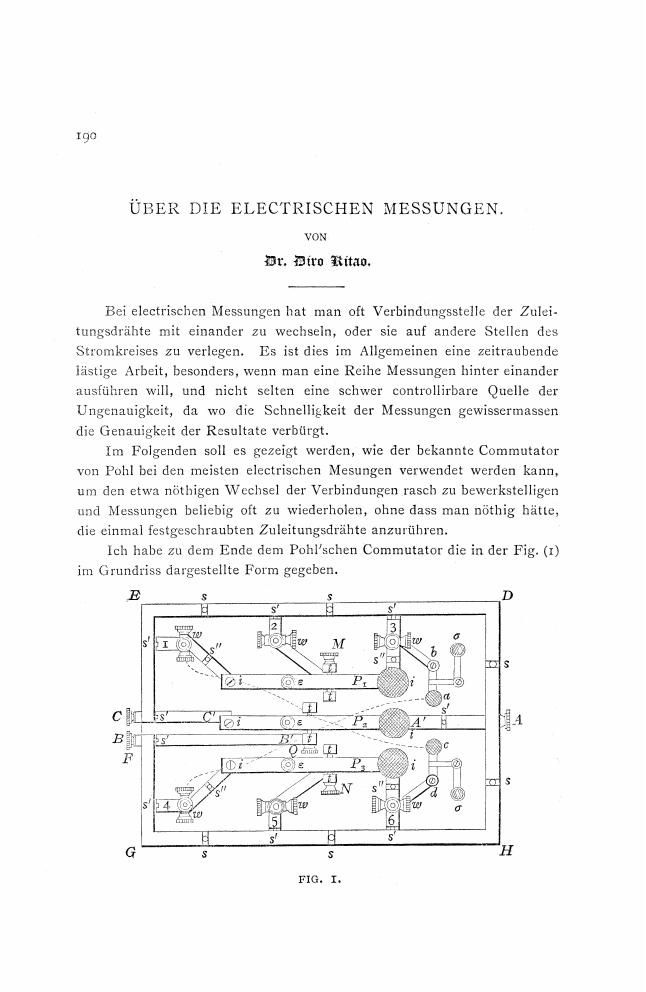
1 0 0 0 OA ÜBER DIE ELECTRISCHEN MESSUNGEN
- 著者
- Diro Ritao
- 出版者
- THE PHYSICAL SOCIETY OF JAPAN , The Mathematical Society of Japan
- 雑誌
- Tokyo Sugaku-Butsurigaku Kwai Kiji (ISSN:21852669)
- 巻号頁・発行日
- vol.5, no.3, pp.190-214, 1894 (Released:2009-06-09)
- 著者
- A. Tanakadale
- 出版者
- THE PHYSICAL SOCIETY OF JAPAN , The Mathematical Society of Japan
- 雑誌
- Tokyo Sugaku-Butsurigaku Kwai Kiji (ISSN:21852669)
- 巻号頁・発行日
- vol.6, no.2, pp.21-38_1, 1895 (Released:2010-03-16)
1 0 0 0 OA SOME EXTENSIONS OF “IWATA'S THEOREM”
- 著者
- T. Hayashi
- 出版者
- THE PHYSICAL SOCIETY OF JAPAN , The Mathematical Society of Japan
- 雑誌
- Tokyo Sugaku-Butsurigaku Kwai Kiji (ISSN:21852669)
- 巻号頁・発行日
- vol.6, no.3, pp.41-51, 1895 (Released:2010-03-16)